Woodworker's Journal summer-2009, страница 57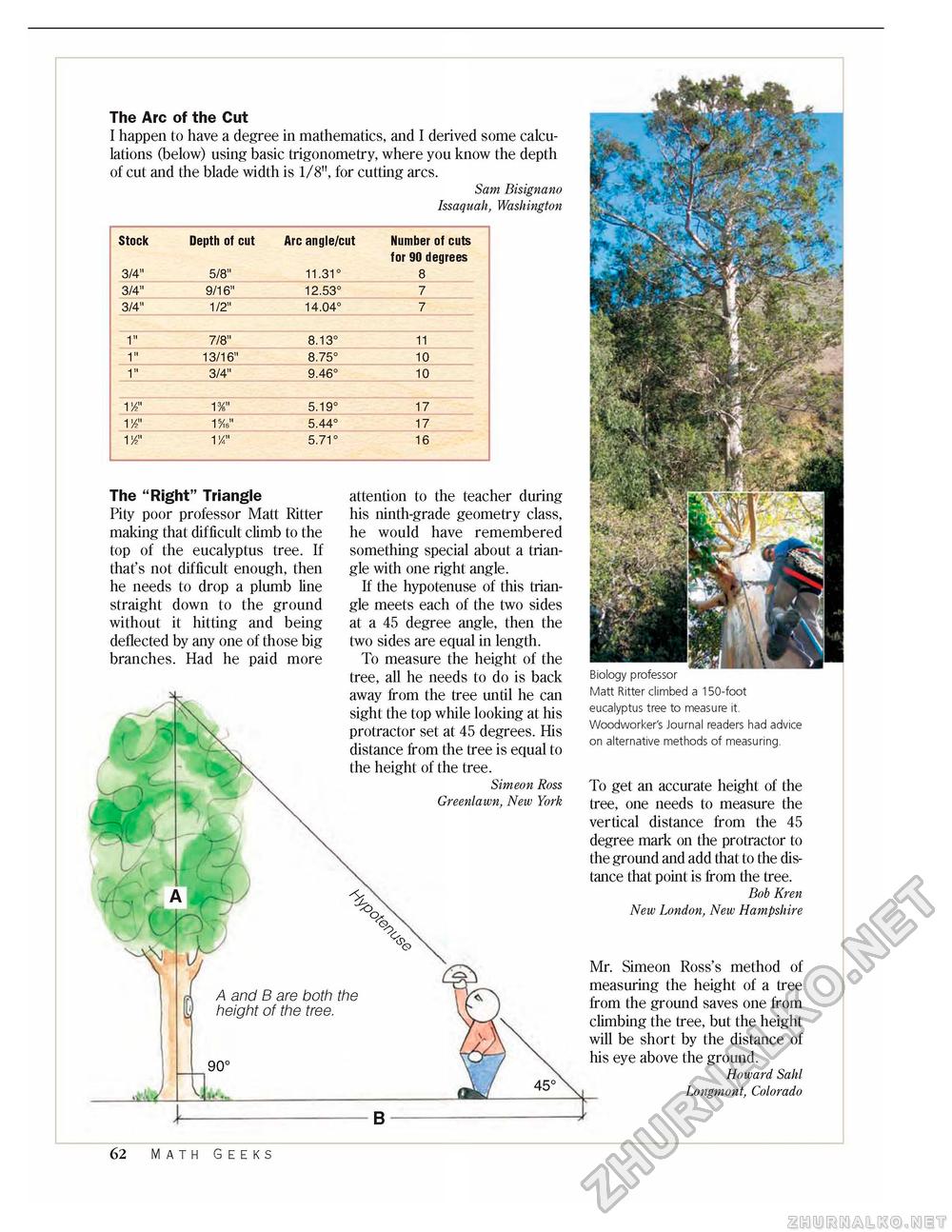

The Arc of the Cut

I happen to have a degree in mathematics, and I derived some calculations (below) using basic trigonometry, where you know the depth of cut and the blade width is 1/8", for cutting arcs.

Sam Bisignano Issaquah, Washington

The "Right" Triangle

Pity poor professor Matt Ritter making that difficult climb to the top of the eucalyptus tree. If that's not difficult enough, then he needs to drop a plumb line straight down to the ground without it hitting and being deflected by any one of those big branches. Had he paid more

attention to the teacher during his ninth-grade geometry class, he would have remembered something special about a triangle with one right angle.

If the hypotenuse of this triangle meets each of the two sides at a 45 degree angle, then the two sides are equal in length.

To measure the height of the tree, all he needs to do is back away from the tree until he can sight the top while looking at his protractor set at 45 degrees. His distance from the tree is equal to the height of the tree.

Simeon Ross Greenlawn, New York

A and B are both the height of the tree.

90°

Biology professor Matt Ritter climbed a 150-foot eucalyptus tree to measure it. Woodworker's Journal readers had advice on alternative methods of measuring.

To get an accurate height of the tree, one needs to measure the vertical distance from the 45 degree mark on the protractor to the ground and add that to the distance that point is from the tree.

Bob Kren

New London, New Hampshire

Mr. Simeon Ross's method of measuring the height of a tree from the ground saves one from climbing the tree, but the height will be short by the distance of his eye above the ground.

Howard Sahl Longmont, Colorado

62 MATH geeks